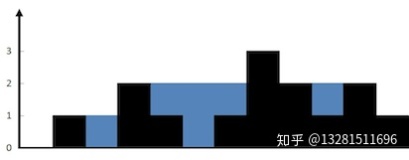
11. Container With Most Water
Problem's Link
----------------------------------------------------------------------------
Mean:
给你一个N条垂直于x轴的直线,从中找两条直线和x轴组成一个桶状容器,使得这个容器的容量最大.
analyse:
1.O(n^2)的做法就不说了,妥妥的超时.
for( int j = i + 1; j <n; ++ j)
...
2.后来在上面方法的基础上加了一些剪枝,但复杂度还是O(n^2),也超时.
class Solution
{
public :
int maxArea( vector < int >& height)
{
if( height . size() <= 1)
return 0;
vector < int > frontIdx;
frontIdx . push_back( 0);
auto ret = 0;
for( auto i = 1; i < height . size(); ++ i)
{
for( auto idx: frontIdx)
{
ret = max( ret , min( height [ idx ], height [ i ]) *( i - idx));
}
auto endIdx =* frontIdx . rbegin();
if( height [ i ] > height [ endIdx ])
frontIdx . push_back( height [ i ]);
}
return ret;
}
};
3.后来看了discuss,看到这个做法,甚是巧妙.
proof:
The O(n) solution with proof by contradiction doesn't look intuitive enough to me. Before moving on, read the algorithm first if you don't know it yet.
Here's another way to see what happens in a matrix representation:
Draw a matrix where the row is the first line, and the column is the second line. For example, say n=6.
In the figures below, x means we don't need to compute the volume for that case: (1) On the diagonal, the two lines are overlapped; (2) The lower left triangle area of the matrix is symmetric to the upper right area.
We start by computing the volume at (1,6), denoted by o. Now if the left line is shorter than the right line, then all the elements left to (1,6) on the first row have smaller volume, so we don't need to compute those cases (crossed by ---).
1 2 3 4 5 6
1 x ------- o
2 x x
3 x x x
4 x x x x
5 x x x x x
6 x x x x x x
Next we move the left line and compute (2,6). Now if the right line is shorter, all cases below(2,6) are eliminated.
1 2 3 4 5 6
1 x ------- o
2 x x o
3 x x x |
4 x x x x |
5 x x x x x |
6 x x x x x x
And no matter how this o path goes, we end up only need to find the max value on this path, which contains n-1 cases.
1 2 3 4 5 6
1 x ------- o
2 x x - o o o
3 x x x o | |
4 x x x x | |
5 x x x x x |
6 x x x x x x
{
public :
int maxArea( vector < int >& height)
{
int si = height . size();
int low = 0 , high = si - 1;
int ret = 0;
while( low < high)
{
ret = max( ret , min( height [ low ], height [ high ]) *( high - low));
if( height [ low ] < height [ high ])
++ low;
else
-- high;
}
return ret;
}
};
Time complexity: O(N)
view code
* -----------------------------------------------------------------
* Copyright (c) 2016 crazyacking.All rights reserved.
* -----------------------------------------------------------------
* Author: crazyacking
* Date : 2016-02-16-09.42
*/
#include <queue>
#include <cstdio>
#include <set>
#include <string>
#include <stack>
#include <cmath>
#include <climits>
#include <map>
#include <cstdlib>
#include <iostream>
#include <vector>
#include <algorithm>
#include <cstring>
using namespace std;
typedef long long( LL);
typedef unsigned long long( ULL);
const double eps( 1e-8);
/*
// Time Limit Exceeded
class Solution
{
public:
int maxArea(vector<int>& height)
{
if(height.size()<=1)
return 0;
vector<int> frontIdx;
frontIdx.push_back(0);
auto ret=0;
for(auto i=1;i<height.size();++i)
{
for(auto idx:frontIdx)
{
ret=max(ret,min(height[idx],height[i])*(i-idx));
}
auto endIdx=*frontIdx.rbegin();
if(height[i]>height[endIdx])
frontIdx.push_back(height[i]);
}
return ret;
}
};
*/
//for(int i=0;i<n;++i)
// for(int j=i+1;j<n;++j)
// ...
class Solution
{
public :
int maxArea( vector < int >& height)
{
int si = height . size();
int low = 0 , high = si - 1;
int ret = 0;
while( low < high)
{
ret = max( ret , min( height [ low ], height [ high ]) *( high - low));
if( height [ low ] < height [ high ])
++ low;
else
-- high;
}
return ret;
}
};
int main()
{
Solution solution;
auto n = 0;
while( cin >>n)
{
vector < int > ve;
for( int i = 0; i <n; ++ i)
{
int tmp;
cin >> tmp;
ve . push_back( tmp);
}
cout << solution . maxArea( ve) << endl;
}
return 0;
}